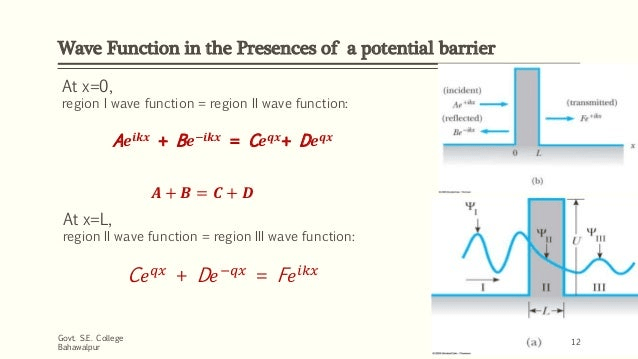
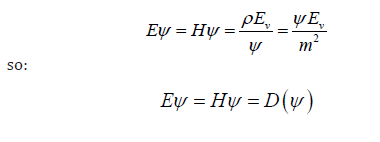(In a more advanced course on quantum mechanics, for example, potential spikes of infinite depth and height are used to model solids). The second condition requires the wave function to be smooth at all points, except in special cases. The first condition avoids sudden jumps or gaps in the wave function. ψ ( x ) ψ ( x ) must not diverge (“blow up”) at x = ± ∞.The first derivative of ψ ( x ) ψ ( x ) with respect to space, d ψ ( x ) / d x d ψ ( x ) / d x, must be continuous, unless V ( x ) = ∞ V ( x ) = ∞.ψ ( x ) ψ ( x ) must be a continuous function.
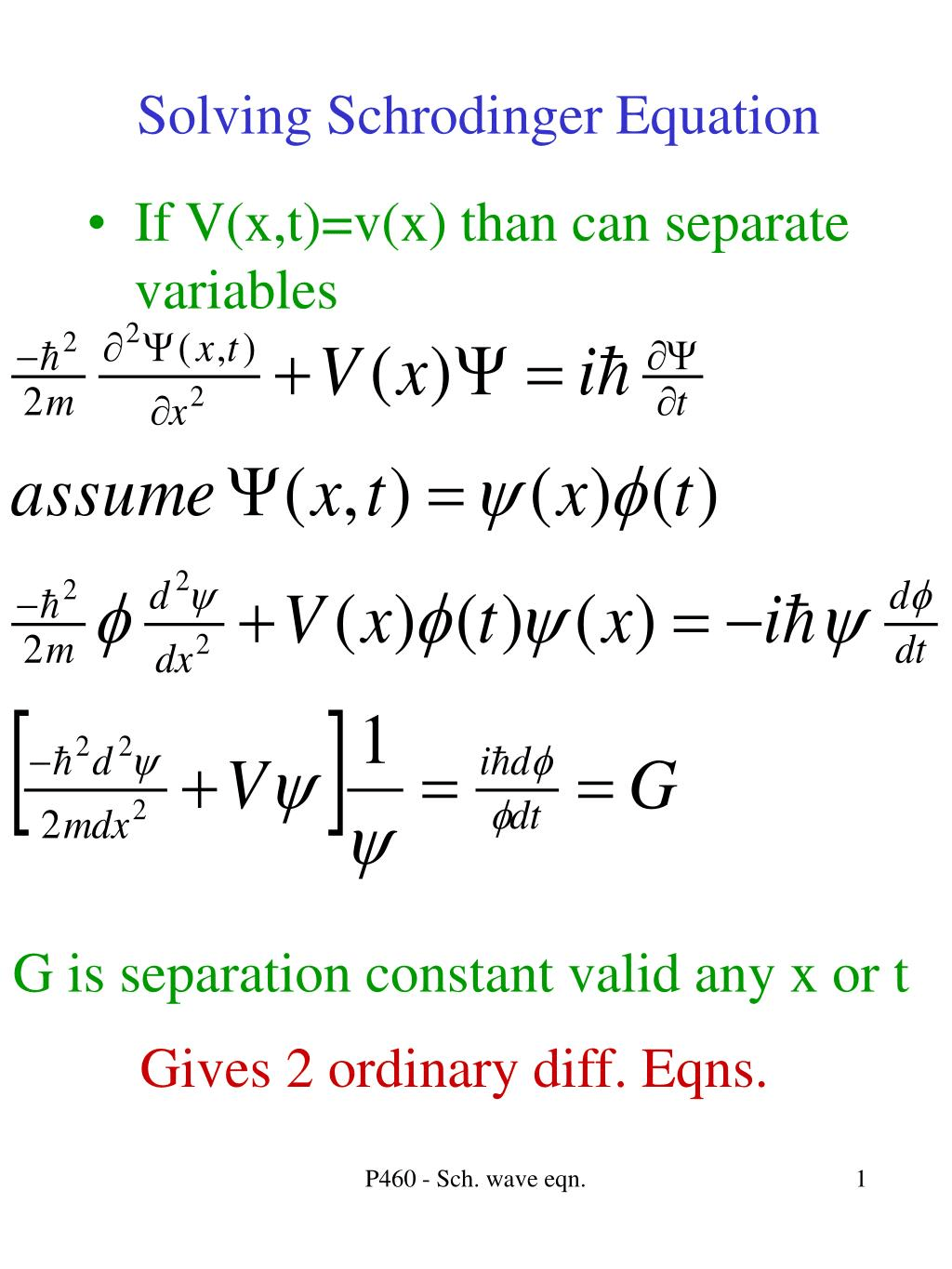
The time-independent wave function ψ ( x ) ψ ( x ) solutions must satisfy three conditions: These cases provide important lessons that can be used to solve more complicated systems. In the next sections, we solve Schrӧdinger’s time-independent equation for three cases: a quantum particle in a box, a simple harmonic oscillator, and a quantum barrier. The wave-function solution to this equation must be multiplied by the time-modulation factor to obtain the time-dependent wave function.
Notice that we use “big psi” ( Ψ ) ( Ψ ) for the time-dependent wave function and “little psi” ( ψ ) ( ψ ) for the time-independent wave function. This equation is called Schrӧdinger’s time-independent equation. Where E is the total energy of the particle (a real number).